Page 105 - Understanding NCERT Science 09

Solution:
Example 8.2 Which would require a
greater force –– accelerating a 2 kg mass From Eq. (8.4) we have m = F/a ; and
1 1
at 5 m s or a 4 kg mass at 2 m s ? m = F/a . Here, a = 10 m s ;
-2
–2
-2
2 2 1
a = 20 m s and F = 5 N.
-2
Solution: 2
-2
Thus, m = 5 N/10 m s = 0.50 kg; and
1
From Eq. (8.4), we have F = ma. m = 5 N/20 m s = 0.25 kg.
-2
2
Here we have m = 2 kg; a = 5 m s -2 If the two masses were tied together,
1 1
-2
and m = 4 kg; a = 2 m s . the total mass, m would be
2 2
-2
Thus, F = m a = 2 kg × 5 m s = 10 N; m = 0.50 kg + 0.25 kg = 0.75 kg.
1 1 1
-2
and F = m a = 4 kg × 2 m s = 8 N. The acceleration, a produced in the
2 2 2
⇒ F > F . combined mass by the 5 N force would
1 2
-2
Thus, accelerating a 2 kg mass at be, a = F/m = 5 N/0.75 kg = 6.67 m s .
-2
5 m s would require a greater force.
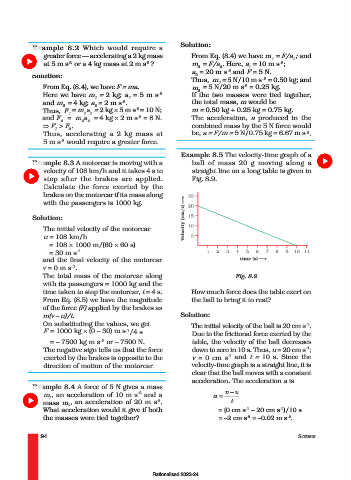
Example 8.5 The velocity-time graph of a
Example 8.3 A motorcar is moving with a ball of mass 20 g moving along a
velocity of 108 km/h and it takes 4 s to straight line on a long table is given in
stop after the brakes are applied. Fig. 8.9.
Calculate the force exerted by the
brakes on the motorcar if its mass along
with the passengers is 1000 kg.
Solution:
The initial velocity of the motorcar
u = 108 km/h
= 108 × 1000 m/(60 × 60 s)
= 30 m s -1
and the final velocity of the motorcar
-1
v = 0 m s .
The total mass of the motorcar along Fig. 8.9
with its passengers = 1000 kg and the
time taken to stop the motorcar, t = 4 s. How much force does the table exert on
From Eq. (8.5) we have the magnitude the ball to bring it to rest?
of the force (F) applied by the brakes as
m(v – u)/t. Solution:
On substituting the values, we get The initial velocity of the ball is 20 cm s .
-1
F = 1000 kg × (0 – 30) m s /4 s Due to the frictional force exerted by the
-1
-2
= – 7500 kg m s or – 7500 N. table, the velocity of the ball decreases
The negative sign tells us that the force down to zero in 10 s. Thus, u = 20 cm s ;
–1
exerted by the brakes is opposite to the v = 0 cm s -1 and t = 10 s. Since the
direction of motion of the motorcar. velocity-time graph is a straight line, it is
clear that the ball moves with a constant
acceleration. The acceleration a is
Example 8.4 A force of 5 N gives a mass
v -u
m , an acceleration of 10 m s and a
–2
1 a =
-2

mass m , an acceleration of 20 m s . t
2
-1
-1
What acceleration would it give if both = (0 cm s – 20 cm s )/10 s
-2
the masses were tied together? = –2 cm s = –0.02 m s .
-2
94 SCIENCE
Rationalised 2023-24