Page 183 - NCERT Science Class 10 English Medium

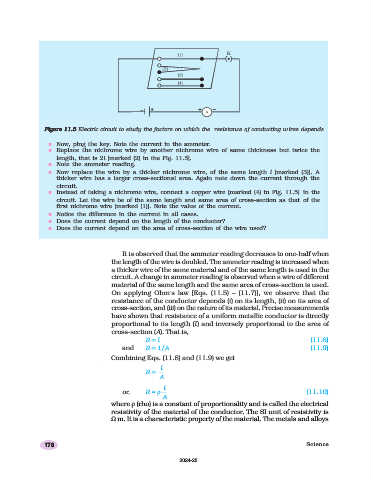
Figure 11.5 Electric circuit to study the factors on which the resistance of conducting wires depends
Figure 11.5
Figure 11.5
Figure 11.5
Figure 11.5
n Now, plug the key. Note the current in the ammeter.
n Replace the nichrome wire by another nichrome wire of same thickness but twice the
length, that is 2l [marked (2) in the Fig. 11.5].
n Note the ammeter reading.
n Now replace the wire by a thicker nichrome wire, of the same length l [marked (3)]. A
thicker wire has a larger cross-sectional area. Again note down the current through the
circuit.
n Instead of taking a nichrome wire, connect a copper wire [marked (4) in Fig. 11.5] in the
circuit. Let the wire be of the same length and same area of cross-section as that of the
first nichrome wire [marked (1)]. Note the value of the current.
n Notice the difference in the current in all cases.
n Does the current depend on the length of the conductor?
n Does the current depend on the area of cross-section of the wire used?

It is observed that the ammeter reading decreases to one-half when
the length of the wire is doubled. The ammeter reading is increased when
a thicker wire of the same material and of the same length is used in the
circuit. A change in ammeter reading is observed when a wire of different
material of the same length and the same area of cross-section is used.
On applying Ohm’s law [Eqs. (11.5) – (11.7)], we observe that the
resistance of the conductor depends (i) on its length, (ii) on its area of
cross-section, and (iii) on the nature of its material. Precise measurements
have shown that resistance of a uniform metallic conductor is directly
proportional to its length (l) and inversely proportional to the area of
cross-section (A). That is,
R ∝ l (11.8)
and R ∝ 1/A (11.9)
Combining Eqs. (11.8) and (11.9) we get

l
R ∝
A
l
or, R = ρ (11.10)
A
where ρ (rho) is a constant of proportionality and is called the electrical
resistivity of the material of the conductor. The SI unit of resistivity is
Ω m. It is a characteristic property of the material. The metals and alloys

178 Science

2024-25